Location Matters, Why Guess?

When developing aerodynamic components, placement matters.  We will prove this point by placing a wing at various heights from the ground and observing the data. Would you be able to guess the height off the ground this wing would make peak downforce? What about peak efficiency?

 We will be viewing CFD images of 4 heights off the ground. However, we ran 30 iterations at a change in distance of 5mm starting at 5mm from the ground. The 4 heights we outline below are 5mm, 35mm, 95mm, and 155mm.

 Let’s look at the 5mm height first. At 5mm off the ground, the flow is choked. Choked flow, in this case, is when the air velocity under the wing will not continue to increase no matter how much more the free-steam velocity increases. This reduction of velocity leads to an increase of pressure which is not what we want when making downforce is key. Looking at the coefficient of pressure plot, note the area of red in front of the airfoil. This is a large stagnation zone, an area of very slow moving air, caused by the airfoil being too close to the ground. The stagnation point is essentially the point with zero velocity.  On the wing, this is located at the very front tip, the dark red location on the cP plot.

Coefficient of Pressure at 5mm

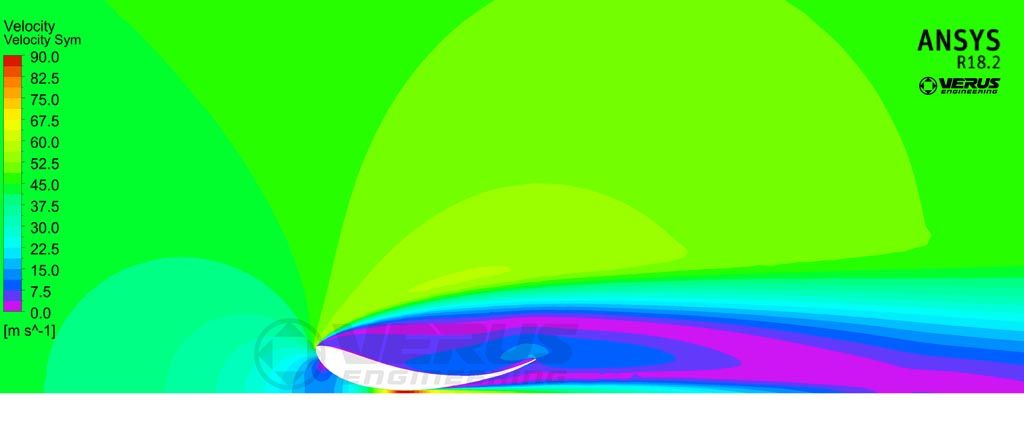
Velocity at 5mm

Moving onto the 35mm height case, both velocity and coefficient of pressure plots look significantly better. The stagnation zone on the Coefficient of Pressure plot has decreased significantly. Also note the larger area of purple below the airfoil (low pressure). Due to the increased height off the ground, more airflow is able to go below the airfoil and generate downforce.

Coefficient of Pressure at 35mm

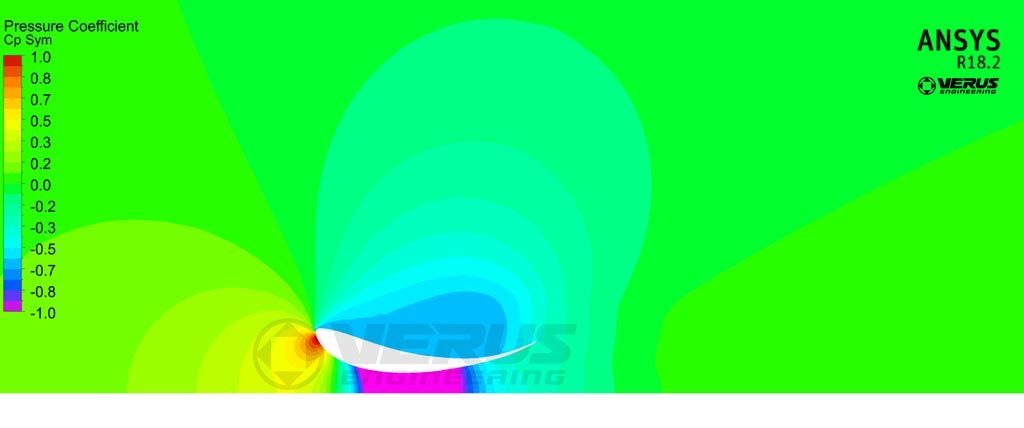
Velocity at 35mm

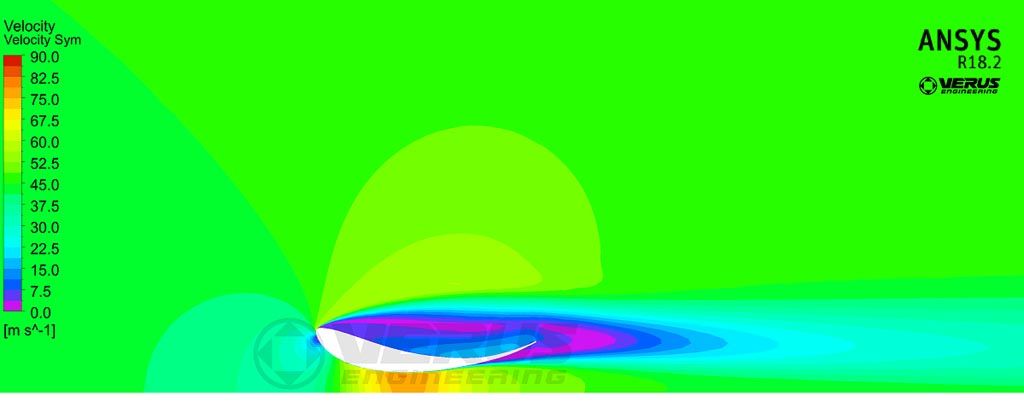
The next height we are viewing is 95 mm above the ground; which is the height the airfoil made maximum downforce. Just because maximum downforce was made at this height, does not mean maximum efficiency is at this height. Note the large low-pressure region under the wing shown in purple plus the decreased high-pressure region around the stagnation point.

Coefficient of Pressure at 95mm

Velocity at 95mm

The last height we will look into is 155mm height. The big item to notice at this height is the decrease of low pressure under the wing compared to the 95mm height. This decrease of the low-pressure zone is due to the wing reacting with the ground. When a wing is close enough to the ground, it makes a converging-diverging “duct” which increases the velocity on the bottom side of the wing, which results in more downforce production.

 Coefficient of Pressure at 155mm

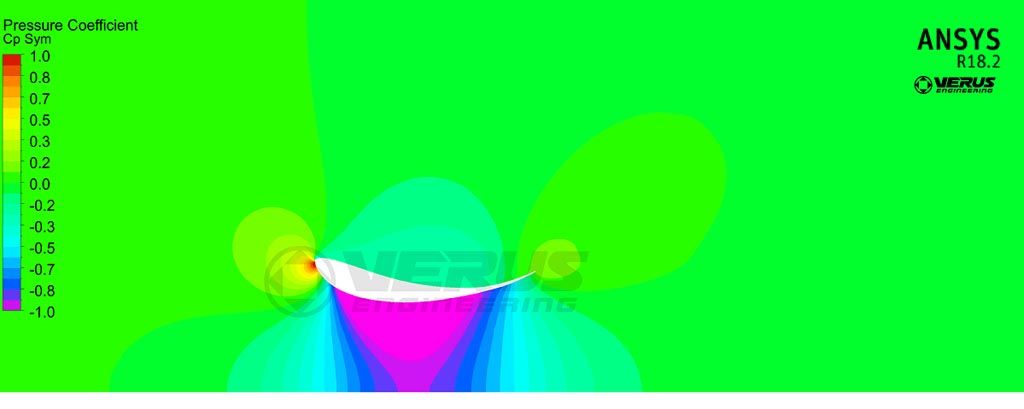
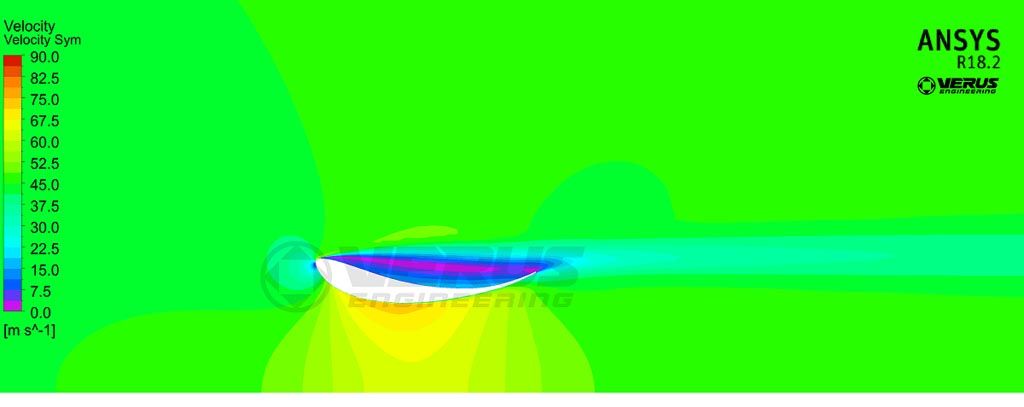
Velocity at 155mm

Now, what does all this mean? We know that downforce production of the wing depends heavily on the height above the ground. If you go too low, the wing actually creates significantly less downforce. We have not discussed drag though. Drag will decrease as wing height is increased until ground effects no longer play a factor. Let’s focus on another important parameter, maximum efficiency. Maximum efficiency will occur when the lift divided by drag is the largest, typically abbreviated L/D.  As long as all the data is available, maximum efficiency is easy to calculate.

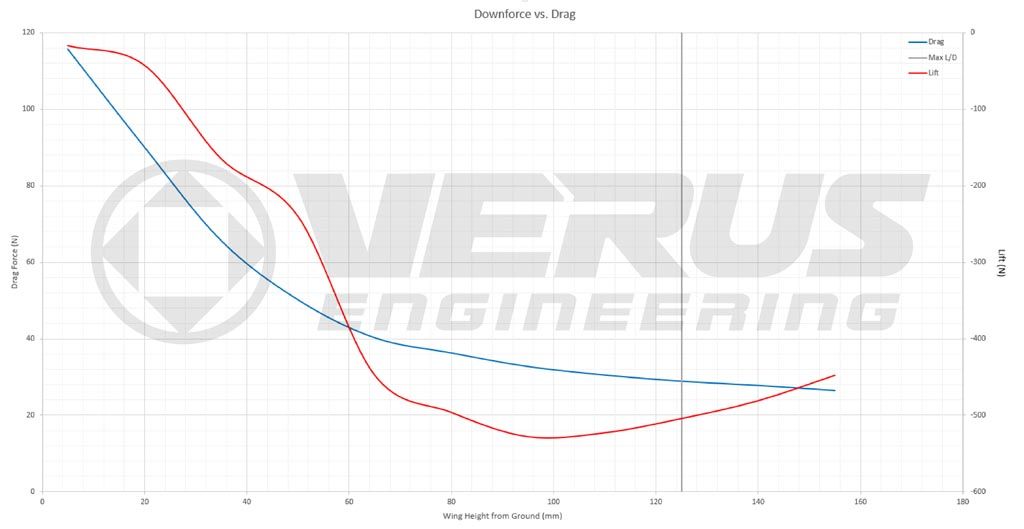
Downforce and Drag as it relates to wing height. Drag forces (N) is on the left while Lift forces are on the right y-axis. The x-axis is the height of the wing above the ground in mm.

 Notice from the graph that downforce increases from 5mm all the way to 95mm; after that point downforce starts to decrease. This decrease in downforce is a result of the ground having a lesser reaction to the wing. Also, note that drag is continually decreasing. Drag and downforce will both normalize and become a constant number. This will occur when free-steam velocity is the only force acting on the wing and the wing is no longer reacting with the ground.

 All of these analyses were done at 45 m/s (100 mph). What would happen when velocity changes?  Or with an object that is not a wing profile?  Or a different AOA?  Don't guess, analyze!